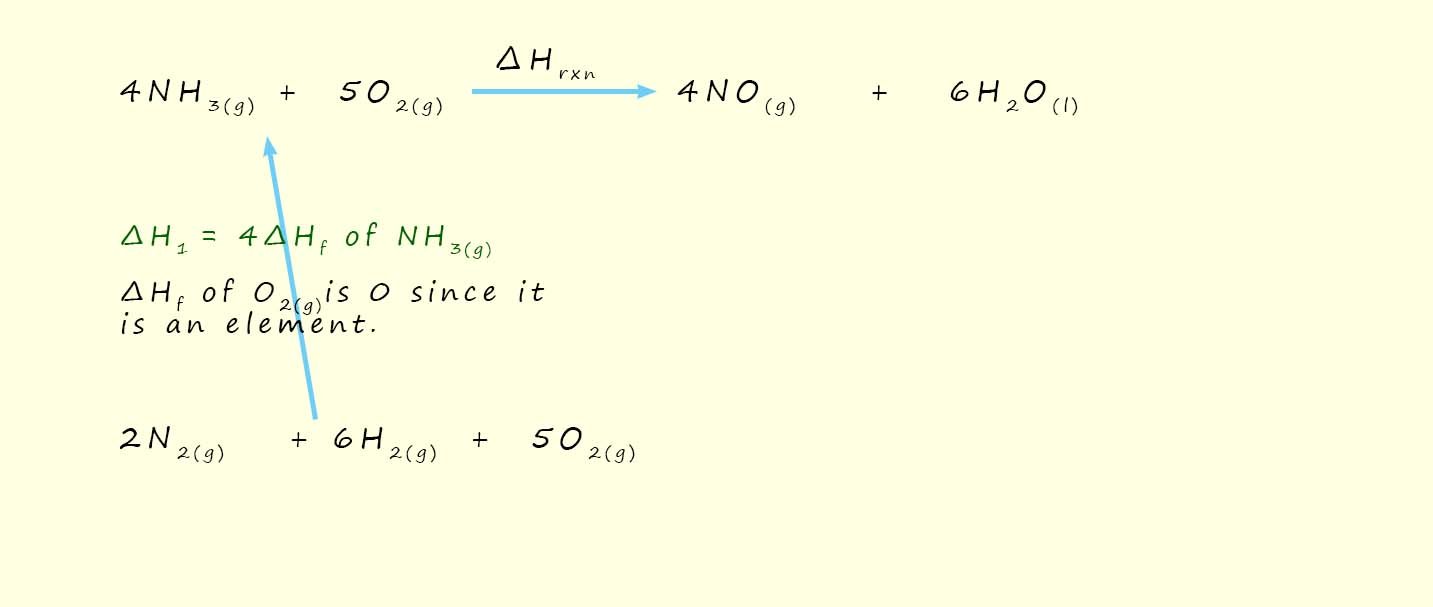Hess's Law

Many chemical reactions are difficult to carry out in the lab under normal conditions. The reasons for this are varied but might include:

The reaction might be very slow and take a long time to complete.
There may be unwanted side reactions taking place

This means that we cannot measure the enthalpy changes for these reactions directly. Luckily we can use Hess's law to calculate the enthalpy changes for these reactions.

Hess's Law is simply an extension of the law of conservation of energy; which states that energy cannot be created or destroyed only change from one form to another. As an example consider the image opposite. Here the change A→B is an exothermic reaction with an enthalpy change (ΔH₁) of -1000kJ.

Now imagine that for some reason the change A→B cannot be carried out directly. However the changes A→C and C→B are possible with enthalpy changes of ΔH₂= -400kJ and ΔH₃= -600kJ. This route from A→B simply goes through an intermediate C. However the important fact is that both reactions, the direct route A→B and the other route through intermediate C both start and end up at the same place. They both start at A and end up at B.

In essence this is Hess's law. Hess's law states that:

The enthalpy change for a reaction depends only on the initial and final states of the reaction and is independent of the route by which the reaction occurs.

So in our example:

The direct route A→B has an enthalpy change of ΔH₁= -1000kJ.
The indirect route A→C has an enthalpy change of ΔH₂= -400kJ and C→B has an enthalpy change of ΔH₃= -600kJ.

Or we could write ΔH₁ = ΔH₂ + ΔH₃.
That is the sum of the two enthalpy changes, ΔH₂ + ΔH₃ is the same as the direct route ΔH₁. Which is just another way of saying that the enthalpy change for a reaction is equal to the sum of the enthalpy changes for any individual steps, as long as you start and end points are the same. That's all there is to Hess's law!

Using enthalpies of combustion (Δ_cH^o) to calculate an enthalpy change

substance	Enthalpy of combustion/kJmol^-1
C_(s)	-394
H_2(g)	-286
CH_4(g)	-890

Example 1 - Calculate the enthalpy of formation of methane (CH_4(g)) using the enthalpies of combustion given in the table opposite.

All the questions you are likely to be asked to solve are all done the same way. So follow the method below and you can't go wrong!

Step 1 - You must write the equation for the reaction you are being asked to calculate the enthalpy change for. In this case we have to calculate the enthalpy of formation of methane. An equation for this enthalpy change is given below:

C_(s) + 2H_2(g) → CH_4(g)

To calculate this enthalpy change we have been given enthalpies of combustion data. To be able to work out the enthalpy change you need the enthalpies of combustion of all the elements and compound present in the equation above; a quick check in the table shows that you have all these enthalpies of combustion. Now all we need to do is draw a simple energy cycle using the data we have. Some students get confused on how to start these cycles, but if you are using enthalpies of combustion; which we are here then the cycle goes "downwards" for the equation above. This is set out below:

Worked example of how to calculate an enthalpy of combustion using enthalpy of formation data.

Methane (CH₄) is a hydrocarbon and it will burn or combust under standard conditions to form 1 mole of carbon dioxide gas and 2 moles of liquid water. Exactly the same as we have for the combustion of the reactants in the above equation. This makes sense since in a chemical reaction all we are in effect doing is rearranging the atoms, no atoms appear or disappear. So for example we have 1 mole of carbon on the reactants side of the above equation, so we will have 1 mole of carbon somewhere on the products side of the equation. The combustion of methane, which we will call ΔH₃ releases 890 kJ of energy. We now have:

Using enthalpies of formation (Δ_fH^o) to calculate an enthalpy change

The other type of problem you are likely to meet is where you have to use enthalpies of formation to calculate an enthalpy change.

substance	Enthalpy of formation/kJmol^-1
NH_3(g)	-46
NO_(g)	90
H₂O_(l)	-286

As before the first step is to write out the equation for the reaction you have been asked to find the enthalpy change for.

Example 2- Calculate the enthalpy change for the following reaction using the enthalpy of formation(Δ_fH^o) data in the table.

4NH_3(g) + 5O_2(g) → 4NO_(g) + 6H₂O_(l)

As before in order to calculate the enthalpy of reaction (Δ_rxnH) using the enthalpy of formation data provided we need to know the enthalpy of formation ( (Δ_fH) for all the substances in our equation. Looking at the table we have all the information we need, remember the enthalpy of formation of an element is by definition 0.
When we drew the energy cycle above using enthalpies of combustion (Δ_cH^o) we drew the cycle "downwards" from our equation. This time using enthalpies of formation (Δ_fH^o) we will work "upwards" towards the equation we have to calculate the enthalpy change for.
So we have:

Step 1:Write the equation you have to calculate the enthalpy change for:

4NH_3(g) + 5O_2(g) → 4NO_(g) + 6H₂O_(l)

Step 2: Start the Hess's law energy cycle by creating an arrow to represent the enthalpy of formation of the reactants (Δ_fH). In this case we only have to do this for the ammonia (NH₃) gas since the other reactant is an element, and the enthalpy of formation of an element (Δ_fH) is always O. You don’t need to write the equation for the Δ_fH of ammonia but here it is anyway!

N_2(g) + 1¹⁄₂H_2(g) → NH_3(g)

enthalpy change

ammonia

₁

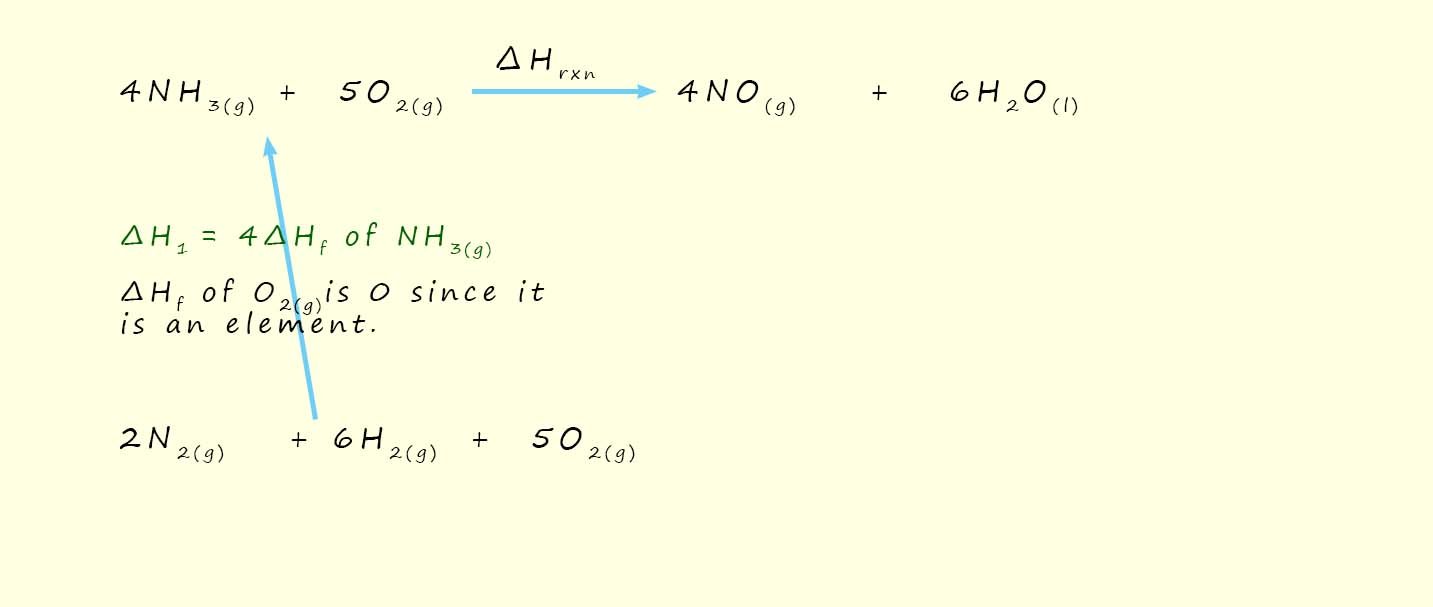
Equation to show enthalpy of formation of a compound

Step 3: As in example 1 - the elements used to form the reactants (2N_2(g) + 6H_2(g) + 5O_2(g) ultimately end up in the products once the chemical reaction is done, after all this is what happens in a chemical reaction, we can show this as:

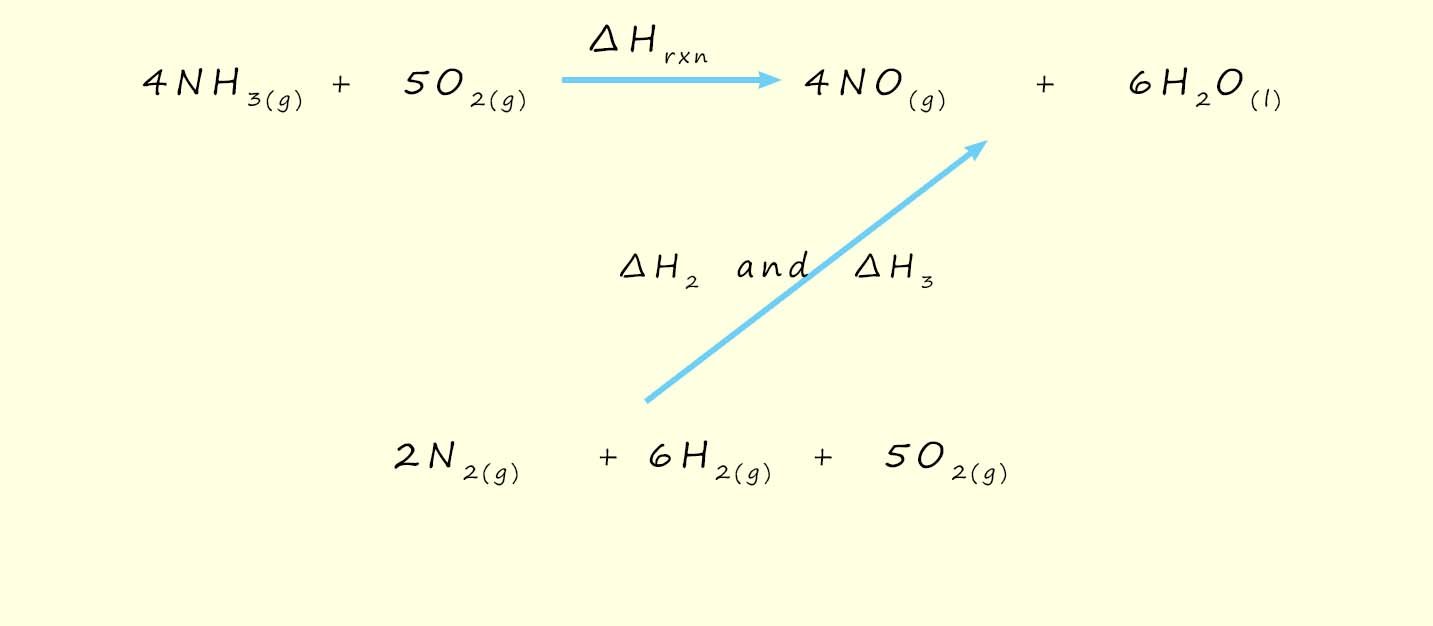
Example on how to Draw a Hess's Law cycle

Now to complete the Hess's Law cycle simply calculate the values for the enthalpy changes when the products form from the reactants as shown in the image above. This time we have to form 4 moles of NO_(g) and 6 moles of water (H₂O_(g)) :
So we have:

¹⁄₂N_2(g) + ¹⁄₂O_2(g)→ NO_(g)

This is the equation for the Δ_fH of NO it will have to be multiplied by 4, since there 4 moles of nitrogen monoxide gas produced. Let's call this enthalpy change ΔH₂. We also form 6 moles of liquid water, an equation to show this enthalpy of formation will be:

H_2(g) + ¹⁄₂ O_2(g)→ H₂O_(l)

This is the equation for the Δ_fH of H₂O it will have to be multiplied by 6. Let's call this enthalpy change ΔH₃

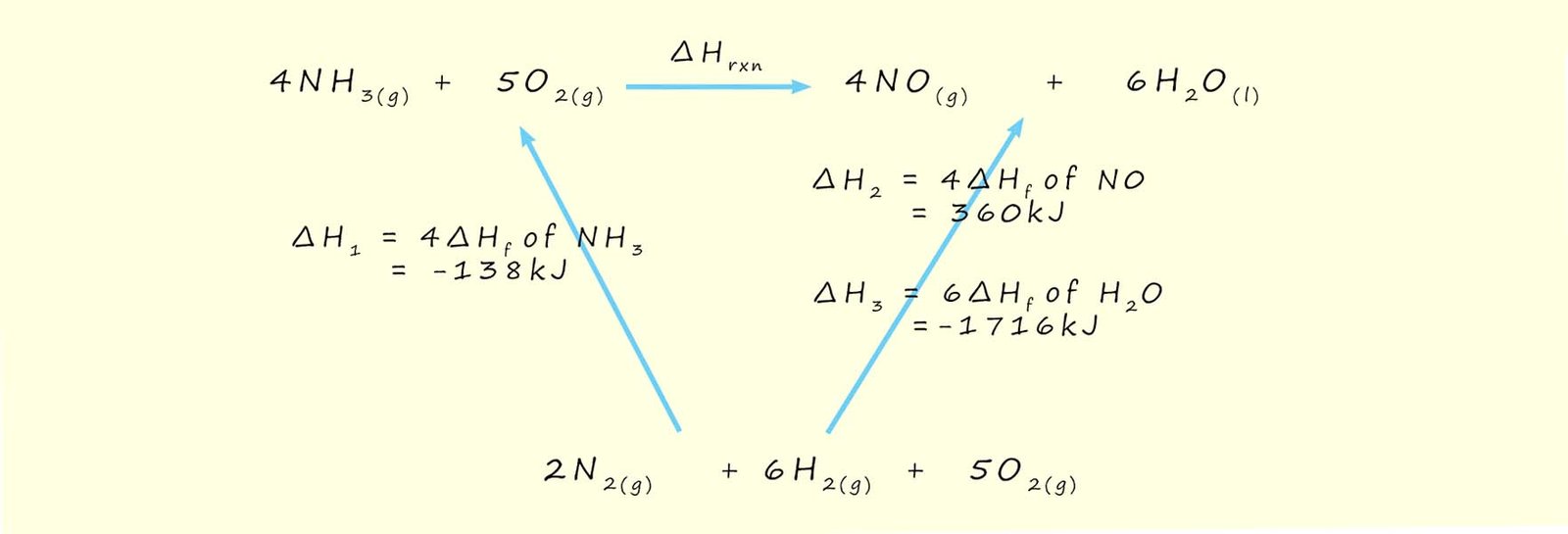
Putting it all together we have: Example of how to draw a Hess's Law cycle using heats of formation

So we can say:

ΔH_rxn= - ΔH₁ + ΔH₂ + ΔH₃
Or
ΔH_rxn = (ΔH₁ + ΔH₂ ) - ΔH₁
=(360 - 1716) - (-138)
= 1218kJ

So to get from the reactants to the products we go against the arrow for ΔH₁ , that is why is appears as -ΔH₁ in the cycle above.

Key Points

The hardest part of getting started with a Hess's law energy cycles is knowing where to start - remember that if you are given enthalpies of combustion data to calculate an unknown enthalpy change your energy cycle goes "downwards" e.g.

Using enthalpies of combustion to calculate the enthalpy change for a reaction (ΔH_rxn)

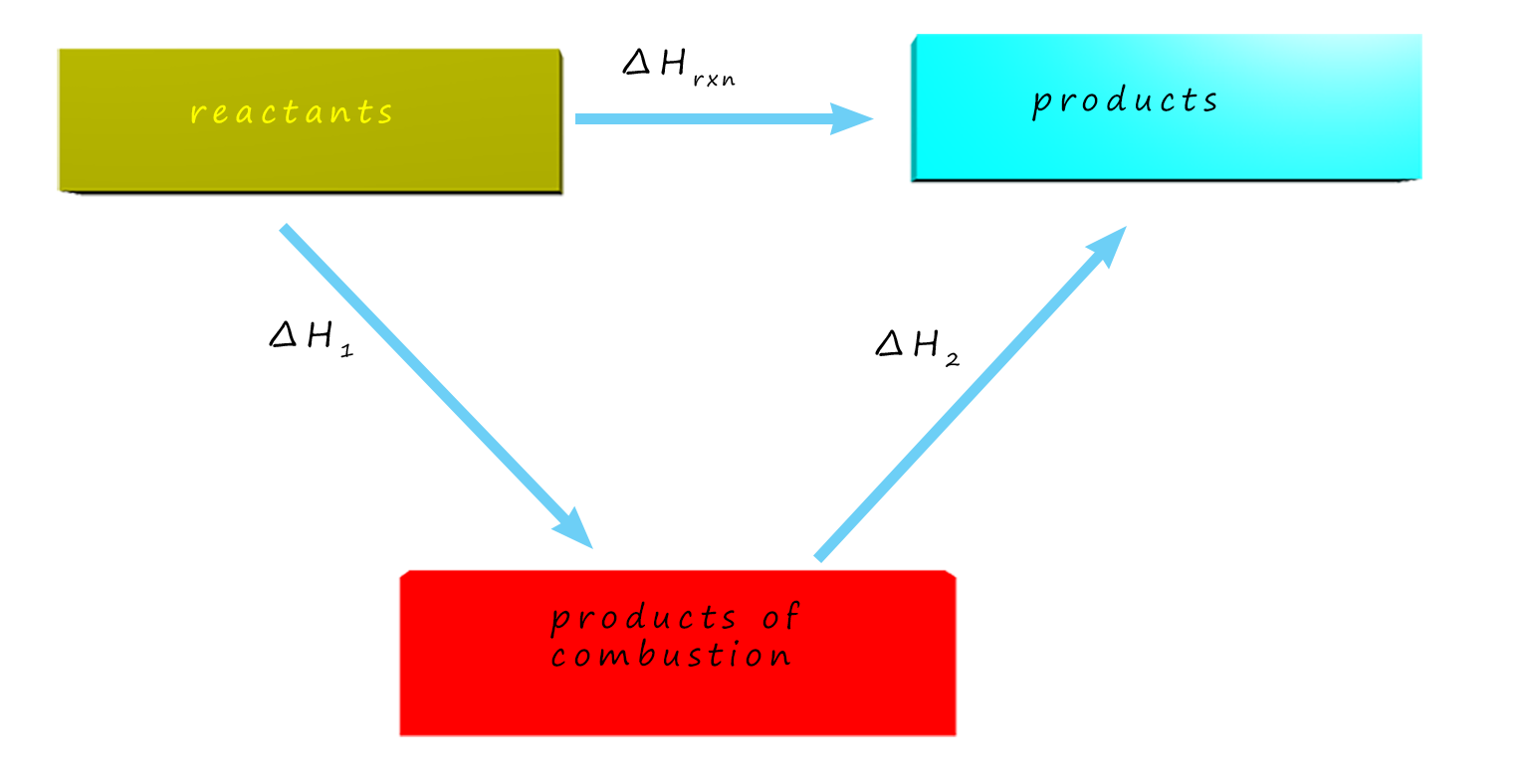
Hess's law cycle showing how to calculate an enthalpy change using enthalpies of combustion data.

Using Hess’s law we can say that: ΔH_rxn = ΔH₁ -ΔH₂

If you are given enthalpies of formation data to calculate an unknown enthalpy change your energy cycle goes "upwards" e.g.

Using enthalpies of formation to calculate the enthalpy change for a reaction (ΔH_rxn)

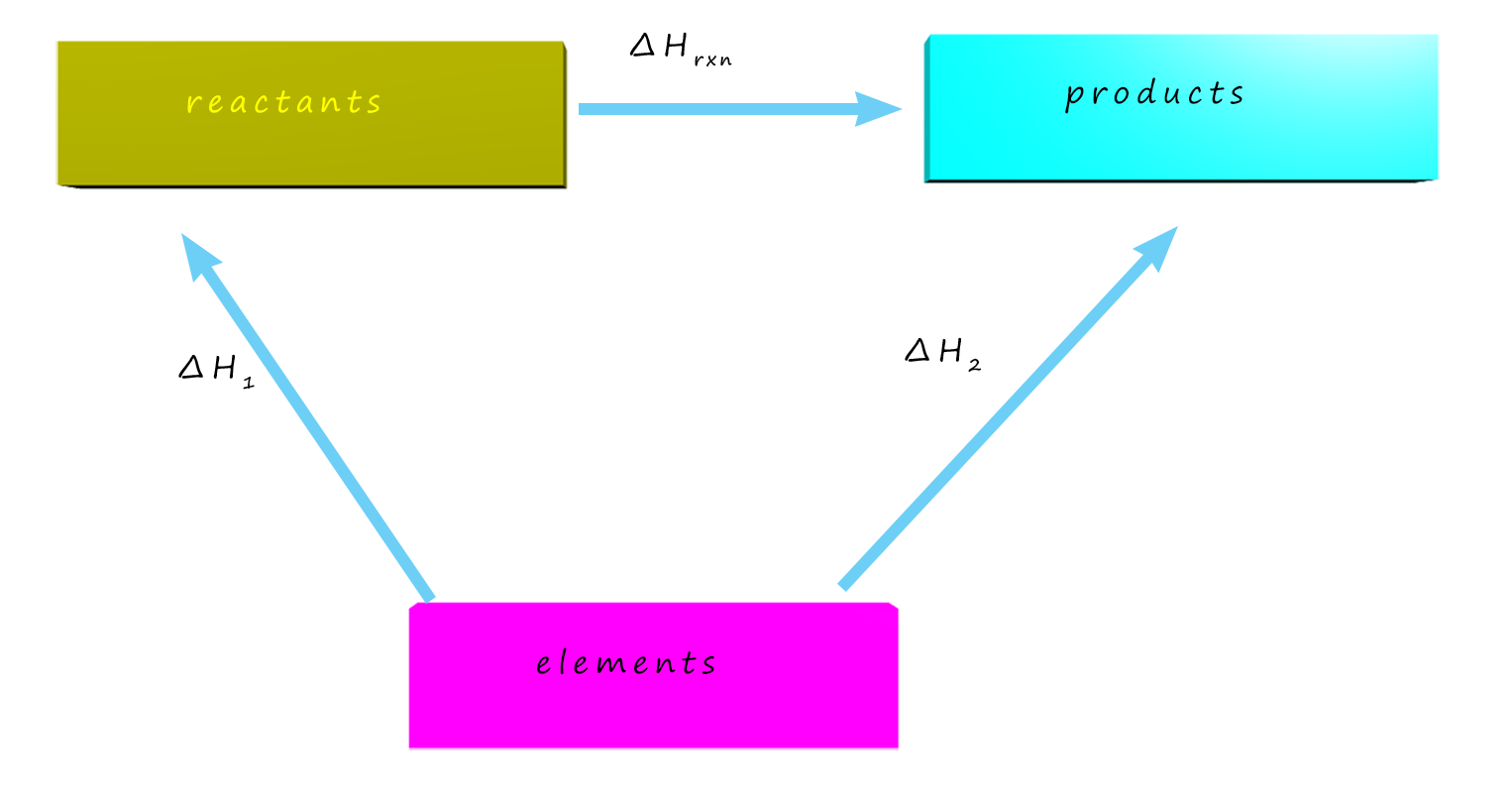
Hess's law cycle showing how to calculate an enthalpy change using enthalpy of formation data.

Using Hess’s law we can say that:

ΔH_rxn = -ΔH₁ + ΔH₂

ΔH_rxn = ΔH₂ - ΔH₁

Hess's Law

Worked examples using Hess' law