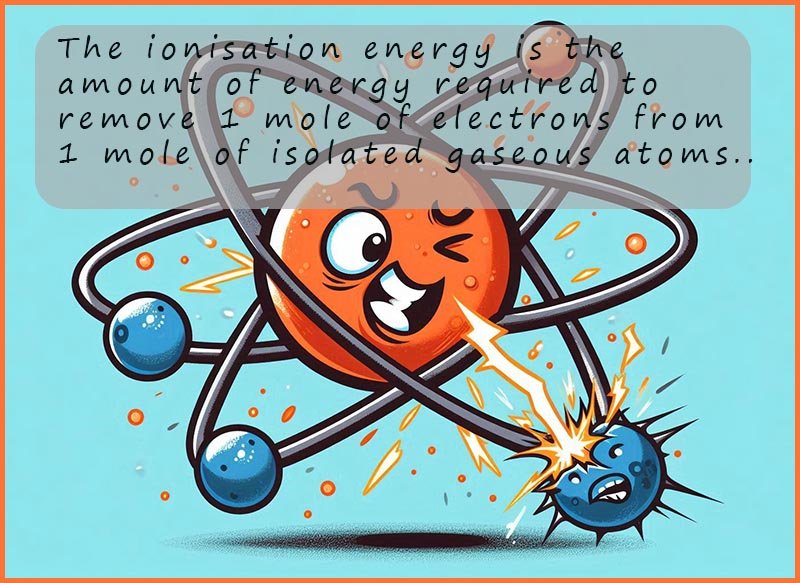
The first ionisation energy is the amount of energy required to remove 1 mole of electrons from an isolated atom in the gaseous state. It can be represented by the equation:
This process will obviously be an endothermic one; energy will have to be provided to remove the electron from the attractive force it feels from the positively charged nucleus. The first ionisation energy varies considerable for different elements. The three factors that you must consider when discussing ionisation energy are:
The first ionisation energies for the elements in group 2 of the periodic table are shown in the bar chart below. The general trend is fairly obvious, as we go down group 2 from the elements beryllium to barium the ionisation energy drops.
To help explain this trend in the ionisation energies we need to consider the electronic configuration for the group 2 elements, these are shown in the table below:
| element | atomic number | electron configuration |
|---|---|---|
| beryllium | 4 | 1s22s2 |
| magnesium | 12 | 1s22s22p63s2 |
| calcium | 20 | 1s22s22p63s23p64s2 |
| strontium | 38 | 1s22s22p63s23p64s23d104p65s2 |
| barium | 56 | 1s22s22p63s23p64s23d104p65s24d105p66s2 |
We can carry out a very rough calculation to get an idea of the actual effective nuclear charge that the valence shell electrons will feel by simply subtracting the number of electrons in the lower electron shells from the nuclear charge e.g. beryllium (Be) has 4 protons, so its nuclear charge is 4+, now beryllium also has 4 electrons in total with an electron configuration of 1s22s2. There are 2 valence electrons in the 2s sub-shell and with these electrons being in the same sub-shell they will shield each other relatively weakly, so we will assume all the shielding comes from the inner 1s2 electrons. These 2 electrons can shield 2 protons. This means that the outer electrons in theory will feel an effective nuclear charge of only 2+. We can carry out a similar calculation for all the group 2 elements, as shown in the table below:
| element | atomic number (nuclear charge) | number of inner screening electrons | number of valence electrons | effective nuclear charge felt by valence electrons |
|---|---|---|---|---|
| beryllium | 4 | 2 | 2 | 2+ |
| magnesium | 12 | 10 | 2 | 2+ |
| calcium | 20 | 18 | 2 | 2+ |
| strontium | 38 | 36 | 2 | 2+ |
| barium | 56 | 54 | 2 | 2+ |
This means that the valence electrons in all the group 2 elements will feel an effective nuclear charge of 2+, but of course as we descend the group the distance from the nucleus to the valence electrons increases greatly, so much less energy will be required to separate the outer valence electrons when they are further from the positively charged nucleus, which means that the ionisation energy will get lower as the atoms in group get larger. We can show this simply as:
It is worth mentioning that this picture of shielding described above is a little simplistic but it can act as a useful starting point, to obtain more accurate values for the shielding effects of electrons within shells and sub-shells a quick internet or Youtube search on Slater values maybe useful, but it is also worth mentioning that knowledge of these values or how to calculate them then are not covered in the A-level specification, but they are easy to use and learn and may provide a better understanding of the shielding effects of electrons.
Ionisation energies are a good source of evidence for the presence of electron shells and sub-shells. So far we have only considered the enthalpy changes for the first ionisation energy of an element:
| ionisation energy | 1st | 2nd | 3rd | 4th | 5th | 6th | 7th |
|---|---|---|---|---|---|---|---|
| energy required/kJmol-1 | 578 | 1820 | 2750 | 11 570 | 14 840 | 18 375 | 23 299 |
As a further example consider the alkali metal sodium, which has an electron configuration of 1s22s22p63s1 .
Sodium has 1 valence electron in the 3s sub-shell. Once this electron
is removed the sodium ion (Na+) formed will have a noble
gas (np6) electron configuration, in this case the noble gas
will be neon. Removal
of a further electron will mean removing an electron
from the
second electron shell, one of the electrons in the 2p sub-level or sub-shell would be removed.
These inner or core electrons are much closer
to the nucleus and will
be much more tightly held by the electrostatic attraction to the positively charged nucleus;
this coupled with the fact that we will be
removing an electron from a smaller positively charged
sodium ion means much more energy will be
needed.
The first ionisation energy of sodium is 496k/mol while the
second ionisation energy is 4560kJ/mol, quite an increase! This large increase in energy would be
good evidence for the existence of electron shells within atoms.
A similar pattern is found with the group II metal magnesium (Mg), which has the electron configuration: 1s2222p63s2. Magnesium has 2 valence electrons in the outer 3s sub-shell. You should be able to predict that removing the first two electrons, that is the valence electrons that would normally be lost in a chemical reaction will require energy. Once these two electrons are lost then magnesium will have a noble gas electron configuration (the same as Neon). However to remove a third electron would involve removing one of the electrons from the second principal energy level, this will require a large increase in energy. The table below give the values for the first three ionisation energies of magnesium. This data provides clear evidence for electron shells. Here we have 2 electrons which are relatively easy to remove followed by a third which requires a huge increase in energy to remove:
| ionisation energy | 1st | 2nd | 3rd |
|---|---|---|---|
| energy required/kJmol-1 | 740 | 1819 | 7737 |