The next main topic after this is one is identifying the trends
and patterns in the ionisation energies of elements across a period and down a group in the periodic table, however to
fully grasp the concepts involved in
identifying the trends and patterns present in ionisation energies
it would be helpful to quickly look at the trends in the size of atoms and ions.
However you might be wondering how it is possible to measure the size of atoms
with any accuracy at all,
since the quantum mechanical model of the atom assumes that the electrons are present in orbitals and an
orbital is a volume of space where there is a high probability
of finding an electron and so we cannot be
100% certain of the size of these orbitals
and there for the size of the atom, and to smudge the issue even more it is not possible to measure the position of the electrons within
these orbitals with any sort of precision due to the uncertainty principle.
Measuring the distance between two nuclei in a solid is a common method used to find the atomic radius of an atom, especially for metals that form crystals with regularly arranged atoms. We can use techniques like X-ray diffraction to measure these distances very precisely. The distance between these bonded nuclei is then divided by two to estimate the radius of a single atom (assuming a spherical shape for simplicity). For non-metals, we can analyse molecules where the atoms are covalently bonded. The bond length, which is half the distance between the nuclei of the bonded atoms, can be measured using spectroscopy techniques like microwave spectroscopy.
What trends might you expect to find in the atomic radius
of atoms if you were to go down a group in the periodic table? Or what about
as we cross a period in the periodic table?
Well take the alkali metals (Li, Na, K, Rb, Cs) in group I of the periodic table as an example, as we
descend group I an extra
electron shell or principal energy level is added for each element.
Li in period 2 has an electronic configuration
of 1s22s1,
Na in period 3,
has an electronic configuration of 1s22s22p63s1, now adding
the extra electron shell as we move down group 1 in the periodic table will increase the atomic radius.
The next element down in period 4 after sodium is potassium; now potassium will have an electron configuration of 1s22s22p63s23p64s1, its outer shell valence
electron is in a 4s sub-shell,
so it will be even larger than sodium. The table below lists the atomic radius for each of the alkali metals up to rubidium and the trend in the atomic radius is as mentioned above; as you descend a group in the periodic table the atoms get larger.
| element | Li | Na | K | Rb |
|---|---|---|---|---|
| atomic radius (pm) | 128 | 166 | 203 | 290 |
| relative sizes of atoms |  |
|
|
 |
Moving down one period in any one particular group in the periodic table to the next period adds an additional electron shell which increases the size of the atom. This is despite the fact that the nuclear charge; that is the number of protons present in the nucleus is increasing as we descend the group which will have the effect of trying to pull in the electrons and decrease the size of the atom, however as the atoms get larger there will be an increase in shielding which will help screen the additional nuclear charge. That is the shielding helps to reduce the effective nuclear charge experienced by the outer valence electrons, mitigating some of the inward pull or attraction towards the nucleus, this is explained in more detail below. So overall the increase in the number of electron shells has a greater influence on the atomic radius compared to the increase in nuclear charge within a group.
To give a simple overview of electron shielding, consider lithium and sodium, alkali metals in group 1 of the periodic table. Lithium has an electron configuration of 1s²2s¹ and has 3 protons in its nucleus. While all the electrons in the lithium atom experience attraction from the positively charged the protons, the two inner (1s) electrons are closer to the nucleus compared to the outer (2s) electron. This proximity allows the inner electrons to partially shield the outer electron from the full positive charge of the nucleus. We express this shielding effect as the concept of effective nuclear charge (Zeff). In this case, the Zeff experienced by the valence electron (2s) in lithium will be slightly less than +3 due to some shielding by the inner electrons. Similarly, sodium (1s²2s²2p⁶3s¹) has 11 electrons. The inner 10 electrons (in the 1s and 2s/2p sub-levels) shield the outermost (3s) electron to a greater extent than in lithium because there are more electrons between the nucleus and the valence electron. This results in a lower Zeff for the valence electron in sodium compared to lithium. However, the increased distance of the valence electron from the nucleus in sodium also weakens the attraction, further contributing to its lower overall attraction to the nucleus. If you would like to have a go at calculating Zeff values a quick search of "Slater values" will set you the right track, however these values are not required in A-level chemistry.
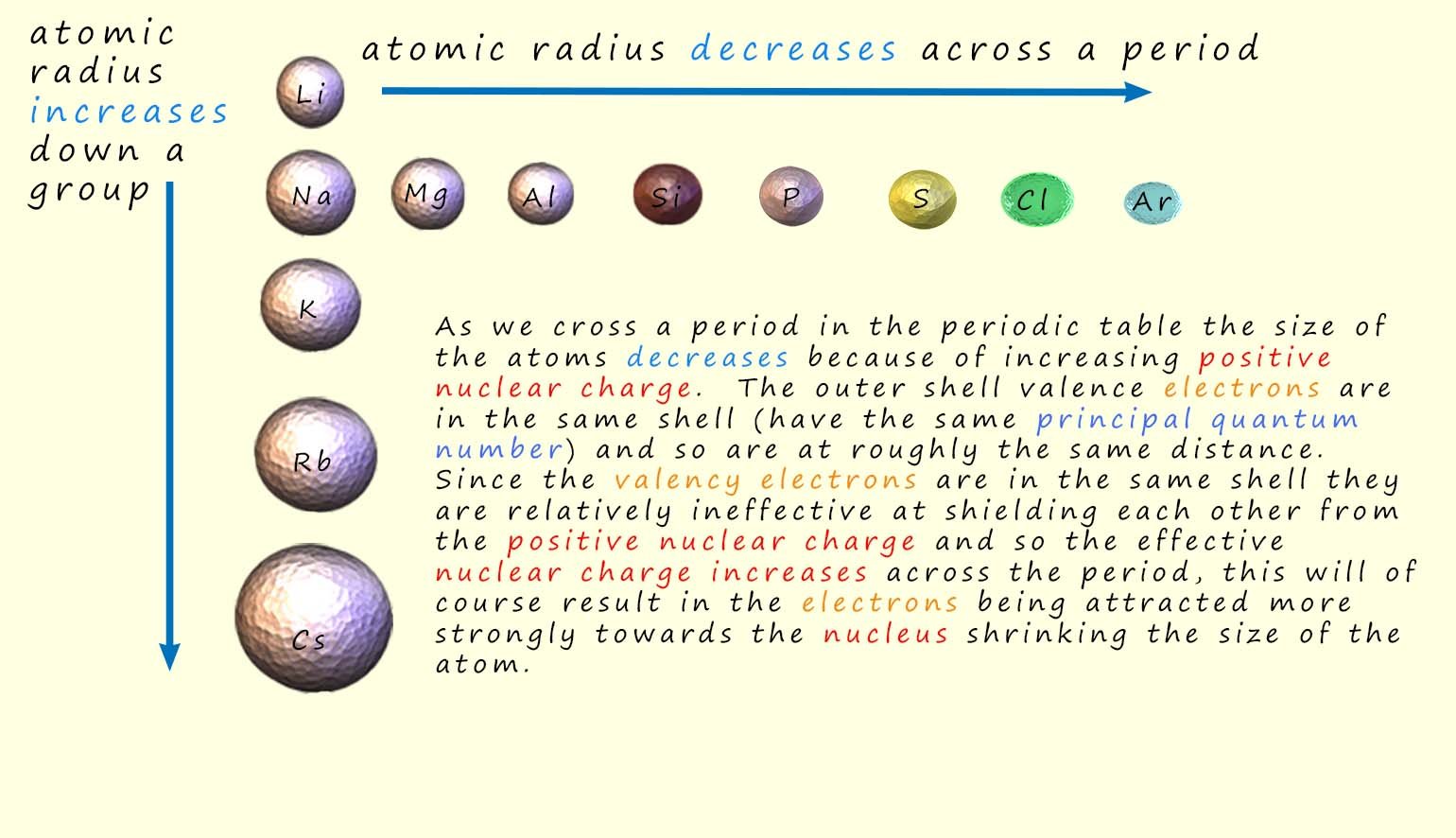
The atoms get smaller as we cross a period in the period table; this is perhaps not what you were expecting! However if you consider the electronic configurations of the elements and the increasing nuclear charge as we cross a period it is an entirely obvious conclusion. The decrease in the size of the atoms is simply because the outer shell valence electrons are all roughly at the same distance from the nucleus since they are in the same principal energy level/sub-level and the ability of electrons to shield each other from the effect of the nuclear charge is reduce by the fact that the valence electrons are all in the same shell. The amount of shielding experienced by an electron depends both on the shell and sub-shell it is in. Generally valance shell electrons are:
Since crossing period 3 the electrons are being added to the 3s and 3p sub-shells they are ineffective at shielding each other from the increasing nuclear charge. So the effective nuclear charge that each electron experiences is increasing so the atoms decrease in size as a result. This is illustrated in the diagram below:
